Past Status (before 2017) | Papers

24

Characterization of Fish Schooling Behavior with Different Numbers of Medaka (Oryzias latipes) and Goldfish (Carassius auratus) Using a Hidden Markov Model

Wonju Jeon (Seung-Ho Kang, Joo-Baek Leem, Sang-Hee Lee) | PHYSICA A 392 (2013)

Fish that swim in schools benefit from increased vigilance, and improved predator recognition and assessment. Fish school size varies according to species and environmental conditions. In this study, we present a Hidden Markov Model (HMM) that we use to characterize fish schooling behavior in different sized schools, and explore how school size affects schooling behavior. We recorded the schooling behavior of Medaka (Oryzias latipes) and goldfish (Carassius auratus) using different numbers of individual fish (10–40), in a circular aquarium. Eight to ten 3 s video clips were extracted from the recordings for each group size. Schooling behavior was characterized by three variables: linear speed, angular speed, and Pearson coefficient. The values of the variables were categorized into two events each for linear and angular speed (high and low), and three events for the Pearson coefficient (high, medium, and low). Schooling behavior was then described as a sequence of 12 events (undefinedundefinedundefinedundefinedundefined2×2×3 ), which was input to an HMM as data for training the model. Comparisons of model output with observations of actual schooling behavior demonstrated that the HMM was successful in characterizing fish schooling behavior. We briefly discuss possible applications of the HMM for recognition of fish species in a school, and for developing bio-monitoring systems to determine water quality. Highlights ？ We used a Hidden Markov Model to describe schooling behavior in common fish species. ？ Model output was consistent with observations of actual fish schooling behavior. ？ HMMs may have applications in field monitoring of fish species and water quality.

초록

Fish that swim in schools benefit from increased vigilance, and improved predator recognition and assessment. Fish school size varies according to species and environmental conditions. In this study, we present a Hidden Markov Model (HMM) that we use to characterize fish schooling behavior in different sized schools, and explore how school size affects schooling behavior. We recorded the schooling behavior of Medaka (Oryzias latipes) and goldfish (Carassius auratus) using different numbers of individual fish (10–40), in a circular aquarium. Eight to ten 3 s video clips were extracted from the recordings for each group size. Schooling behavior was characterized by three variables: linear speed, angular speed, and Pearson coefficient. The values of the variables were categorized into two events each for linear and angular speed (high and low), and three events for the Pearson coefficient (high, medium, and low). Schooling behavior was then described as a sequence of 12 events ( $2 \times 2 \times 3$ ), which was input to an HMM as data for training the model. Comparisons of model output with observations of actual schooling behavior demonstrated that the HMM was successful in characterizing fish schooling behavior. We briefly discuss possible applications of the HMM for recognition of fish species in a school, and for developing bio-monitoring systems to determine water quality.

Highlights

？ We used a Hidden Markov Model to describe schooling behavior in common fish species. ？ Model output was consistent with observations of actual fish schooling behavior. ？ HMMs may have applications in field monitoring of fish species and water quality.

초록

Fish that swim in schools benefit from increased vigilance, and improved predator recognition and assessment. Fish school size varies according to species and environmental conditions. In this study, we present a Hidden Markov Model (HMM) that we use to characterize fish schooling behavior in different sized schools, and explore how school size affects schooling behavior. We recorded the schooling behavior of Medaka (Oryzias latipes) and goldfish (Carassius auratus) using different numbers of individual fish (10–40), in a circular aquarium. Eight to ten 3 s video clips were extracted from the recordings for each group size. Schooling behavior was characterized by three variables: linear speed, angular speed, and Pearson coefficient. The values of the variables were categorized into two events each for linear and angular speed (high and low), and three events for the Pearson coefficient (high, medium, and low). Schooling behavior was then described as a sequence of 12 events ( $2 \times 2 \times 3$ ), which was input to an HMM as data for training the model. Comparisons of model output with observations of actual schooling behavior demonstrated that the HMM was successful in characterizing fish schooling behavior. We briefly discuss possible applications of the HMM for recognition of fish species in a school, and for developing bio-monitoring systems to determine water quality.

Highlights

？ We used a Hidden Markov Model to describe schooling behavior in common fish species. ？ Model output was consistent with observations of actual fish schooling behavior. ？ HMMs may have applications in field monitoring of fish species and water quality.

More

23

Some Identities on the Generalized q-Bernoulli, q-Euler and q-Genocchi Polynomials

Daeyeoul Kim (Burak Kurt, Veli Kurt) | Abstract and Applied Analysis 2013 (2013)

Mahmudov (2012, 2013) introduced and investigated some q q -extensions of the q q -Bernoulli polynomials B (α) n,q (x,y) ？n,qαx,y of order α α , the q q -Euler polynomials E (α) n,q (x,y) ？n,qαx,y of order α α , and the q q -Genocchi polynomials G (α) n,q (x,y) ？？n,qαx,y of order α α . In this paper, we give some identities for B (α) n,q (x,y) ？n,qαx,y , G (α) n,q (x,y) ？？n,qαx,y , and E (α) n,q (x,y) ？n,qαx,y and the recurrence relations between these polynomials. This is an analogous result to the q q -extension of the Srivastava-Pintér addition theorem in Mahmudov (2013).

초록

Mahmudov (2012, 2013) introduced and investigated some $q$ -extensions of the $q$ -Bernoulli polynomials ${\scr B}_{n,q}^{(\alpha )}(x,y)$ of order $\alpha$ , the $q$ -Euler polynomials ${\scr E}_{n,q}^{(\alpha )}(x,y)$ of order $\alpha$ , and the $q$ -Genocchi polynomials ${\mathrm{\scr G}}_{n,q}^{(\alpha )}(x,y)$ of order $\alpha$ . In this paper, we give some identities for ${\scr B}_{n,q}^{(\alpha )}(x,y)$ , ${\mathrm{\scr G}}_{n,q}^{(\alpha )}(x,y)$ , and ${\scr E}_{n,q}^{(\alpha )}(x,y)$ and the recurrence relations between these polynomials. This is an analogous result to the $q$ -extension of the Srivastava-Pintér addition theorem in Mahmudov (2013).

초록

Mahmudov (2012, 2013) introduced and investigated some $q$ -extensions of the $q$ -Bernoulli polynomials ${\scr B}_{n,q}^{(\alpha )}(x,y)$ of order $\alpha$ , the $q$ -Euler polynomials ${\scr E}_{n,q}^{(\alpha )}(x,y)$ of order $\alpha$ , and the $q$ -Genocchi polynomials ${\mathrm{\scr G}}_{n,q}^{(\alpha )}(x,y)$ of order $\alpha$ . In this paper, we give some identities for ${\scr B}_{n,q}^{(\alpha )}(x,y)$ , ${\mathrm{\scr G}}_{n,q}^{(\alpha )}(x,y)$ , and ${\scr E}_{n,q}^{(\alpha )}(x,y)$ and the recurrence relations between these polynomials. This is an analogous result to the $q$ -extension of the Srivastava-Pintér addition theorem in Mahmudov (2013).

More

22

A Diophantine problem concerning polygonal numbers

Daeyeoul Kim (Akos Pinter,Yoon Kyung Park) | Bulletin of the Australian Mathematical Society 88 (2013)

Motivated by some earlier Diophantine works on triangular numbers by Ljunggren and Cassels, we consider similar problems for general polygonal numbers.

More

21

Competitively Tight Graphs

Suh-Ryung Kim (Yoshio Sano, Boram Park, Jung Yeun Lee) | Annals of Combinatorics 17 (2013)

The competition graph of a digraph D is a (simple undirected) graph which has the same vertex set as D and has an edge between two distinct vertices x and y if and only if there exists a vertex v in D such that (x, v) and (y, v) are arcs of D. For any graph G, G together with sufficiently many isolated vertices is the competition graph of some acyclic digraph. The competition number k(G) of a graph G is the smallest number of such isolated vertices. Computing the competition number of a graph is an NP-hard problem in general and has been one of the important research problems in the study of competition graphs. Opsut [1982] showed that the competition number of a graph G is related to the edge clique cover number θ E (G) of the graph G via θ E (G) − |V(G)| + 2 ≤ k(G) ≤ θ E (G). We first show that for any positive integer m satisfying 2 ≤ m ≤ |V(G)|, there exists a graph G with k(G) = θ E (G) − |V(G)| + m and characterize a graph G satisfying k(G) = θ E (G). We then focus on what we call competitively tight graphs G which satisfy the lower bound, i.e., k(G) = θ E (G) − |V(G)| + 2. We completely characterize the competitively tight graphs having at most two triangles. In addition, we provide a new upper bound for the competition number of a graph from which we derive a sufficient condition and a necessary condition for a graph to be competitively tight.

초록

The competition graph of a digraph D is a (simple undirected) graph which has the same vertex set as D and has an edge between two distinct vertices x and y if and only if there exists a vertex v in D such that (x, v) and (y, v) are arcs of D. For any graph G, G together with sufficiently many isolated vertices is the competition graph of some acyclic digraph. The competition number k(G) of a graph G is the smallest number of such isolated vertices. Computing the competition number of a graph is an NP-hard problem in general and has been one of the important research problems in the study of competition graphs. Opsut [1982] showed that the competition number of a graph G is related to the edge clique cover number θ _E(G) of the graph G via θ _E(G) − |V(G)| + 2 ≤ k(G) ≤ θ _E(G). We first show that for any positive integer m satisfying 2 ≤ m ≤ |V(G)|, there exists a graph G with k(G) = θ _E(G) − |V(G)| + m and characterize a graph G satisfying k(G) = θ _E(G). We then focus on what we call competitively tight graphs G which satisfy the lower bound, i.e., k(G) = θ _E(G) − |V(G)| + 2. We completely characterize the competitively tight graphs having at most two triangles. In addition, we provide a new upper bound for the competition number of a graph from which we derive a sufficient condition and a necessary condition for a graph to be competitively tight.

초록

The competition graph of a digraph D is a (simple undirected) graph which has the same vertex set as D and has an edge between two distinct vertices x and y if and only if there exists a vertex v in D such that (x, v) and (y, v) are arcs of D. For any graph G, G together with sufficiently many isolated vertices is the competition graph of some acyclic digraph. The competition number k(G) of a graph G is the smallest number of such isolated vertices. Computing the competition number of a graph is an NP-hard problem in general and has been one of the important research problems in the study of competition graphs. Opsut [1982] showed that the competition number of a graph G is related to the edge clique cover number θ _E(G) of the graph G via θ _E(G) − |V(G)| + 2 ≤ k(G) ≤ θ _E(G). We first show that for any positive integer m satisfying 2 ≤ m ≤ |V(G)|, there exists a graph G with k(G) = θ _E(G) − |V(G)| + m and characterize a graph G satisfying k(G) = θ _E(G). We then focus on what we call competitively tight graphs G which satisfy the lower bound, i.e., k(G) = θ _E(G) − |V(G)| + 2. We completely characterize the competitively tight graphs having at most two triangles. In addition, we provide a new upper bound for the competition number of a graph from which we derive a sufficient condition and a necessary condition for a graph to be competitively tight.

More

20

New approach to twisted q-Bernoulli polynomials

Daeyeoul Kim (Yoon Kyung Park, JaKyeong Koo) | Advances in Difference Equations 298 (2013)

By using the theory of basic hypergeometric series, we present some formulas for q-consecutive integers, and we find certain new identities for twisted q-Bernoulli polynomials and q-consecutive integers (Simsek in Adv. Stud. Contemp. Math. 16(2):251-278, 2008). MSC: 11B68, 05A30.

More

19

Certain combinatoric Bernoulli polynomials and convolution sums of divisor functions

Daeyeoul Kim(Nazli Ikikardes) | Advances in Difference Equations 310 (2013)

More

18

Bernoulli numbers and certain convolution sums with divisor functions

Daeyeoul Kim (Nazli Ikikardes, Aeran Kim) | Advances in Difference Equations 277 (2013)

More

17

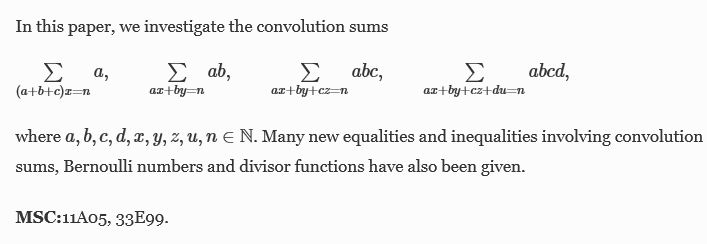
Evaluation of a certain combinatorial convolution sum in higher level cases

Bumkyu Cho(Daeyeoul Kim, Ho Park) | Journal of Mathematical Analysis and Applications 406 (2013)

It is known that certain combinatorial convolution sums involving divisor functions of “levels” 1 and 2 can be explicitly expressed as a linear combination of divisor functions. In this article we deal with a case for arbitrary level and obtain an explicit expression.

More

16

Some Symmetric Identities On Higher Order q-Euler Polynomials And Multivariate p-adic Fermionic q-integral On Zp

Daeyeoul Kim (Minsoo Kim) | Applied Mathematics and Computation 221 (2013)

In this paper we derive some identities of symmetry related to higher order q-Euler polynomials by using the multivariate fermionic p-adic q-integral on undefinedundefinedZp . Furthermore, some of these identities are also related to the q-analogue of the alternating power sums and the multiplication theorem.

초록

In this paper we derive some identities of symmetry related to higher order q-Euler polynomials by using the multivariate fermionic p-adic q-integral on $Z_{p}$ . Furthermore, some of these identities are also related to the q-analogue of the alternating power sums and the multiplication theorem.

초록

In this paper we derive some identities of symmetry related to higher order q-Euler polynomials by using the multivariate fermionic p-adic q-integral on $Z_{p}$ . Furthermore, some of these identities are also related to the q-analogue of the alternating power sums and the multiplication theorem.

More

15

Convolution sums and their relations to Eisenstein series

Daeyeoul Kim (Aeran Kim, Sankaranarayanan Ayyadurai) | Bulletin of the Korean Mathematical Society 50 (2013)

In this paper, we consider several convolution sums, namely, Ai(m,n;N)Ai(m,n;N) (i=1,2,3,4i=1,2,3,4 ), Bj(m,n;N)Bj(m,n;N) (j=1,2,3j=1,2,3 ), and Ck(m,n;N)Ck(m,n;N) (k=1,2,3,？,12k=1,2,3,？,12 ), and establish certain identities involving their finite products. Then we extend these types of product convolution identities to products involving Faulhaber sums. As an application, an identity involving the Weierstrass ℘℘ -function, its derivative and certain linear combination of Eisenstein series is established.

초록

In this paper, we consider several convolution sums, namely, $A_{i} (m, n; N)$ ( $i = 1, 2, 3, 4$ ), $B_{j} (m, n; N)$ ( $j = 1, 2, 3$ ), and $C_{k} (m, n; N)$ ( $k = 1, 2, 3, \dots, 12$ ), and establish certain identities involving their finite products. Then we extend these types of product convolution identities to products involving Faulhaber sums. As an application, an identity involving the Weierstrass $℘$ -function, its derivative and certain linear combination of Eisenstein series is established.

초록

In this paper, we consider several convolution sums, namely, $A_{i} (m, n; N)$ ( $i = 1, 2, 3, 4$ ), $B_{j} (m, n; N)$ ( $j = 1, 2, 3$ ), and $C_{k} (m, n; N)$ ( $k = 1, 2, 3, \dots, 12$ ), and establish certain identities involving their finite products. Then we extend these types of product convolution identities to products involving Faulhaber sums. As an application, an identity involving the Weierstrass $℘$ -function, its derivative and certain linear combination of Eisenstein series is established.

More