Numerical modeling for 3D acoustic wave equation in the frequency domain
Taeyoung Ha, Yunseok Choi, Changsoo Shin, Dong-Joo Min
|
Journal of Seismic Exploration
18
(2009)
Ha, T., Choi, Y., Shin, C.S. and Min, D.-J., 2009. Numerical modeling for 3D acoustic wave
equation in the frequency domain. Joumal of Seisnic Exploration, l8t 57-19.
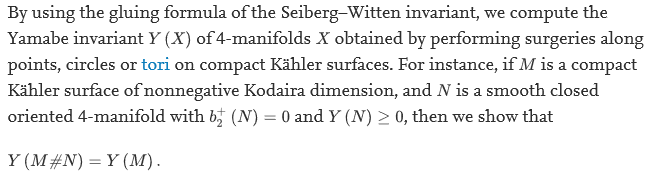
We investigatea frequency-domainfi nite-elementm ethod for three-dimensionaml odeling
of the acousticw ave equation.F requency-domaimn odelingh ass everala dvantageos ver time-domain
modeling,e ven though it requiresh ugec omputationaml emoryc omparedt o time-domainm odeling.
One of these advantages is that multi-shot modeling can be performed more efficiently in the
frequency domain than in the time domain, and another is the ability to work on a
frequency-by-frequencbya sis,w hich makesi t possiblet o distributef requenciesa crossp rocessors.
Consideringt hat frequency-domainm odeling is popular in waveform inversionb ecauseo f source
wavelet estimationa nd multi-shot modeling, 3D frequency-domainfi nite-elementm odelingc an be
effectively used in 3D waveform inversion. We derive a numerical dispersion relationship for the
3D frequency-domainfi nite-elementm ethoda nd then analyzen umericald ispersiono n the basiso f
dispersion curves. From the dispersion analysis, we determine th€ minimum number of grid points
per wavelength. The validity of the 3D finite-element modelirtg algorithm is examined for a
three-layeredm odel and the SEG/EACE salt model.
KEY WORDS:3D modeling, acousticw ave equation,f requency-domainf,i nite-elemem,
numerical dispersion
- 초록
Ha, T., Choi, Y., Shin, C.S. and Min, D.-J., 2009. Numerical modeling for 3D acoustic wave
equation in the frequency domain. Joumal of Seisnic Exploration, l8t 57-19.
We investigatea frequency-domainfi nite-elementm ethod for three-dimensionaml odeling
of the acousticw ave equation.F requency-domaimn odelingh ass everala dvantageos ver time-domain
modeling,e ven though it requiresh ugec omputationaml emoryc omparedt o time-domainm odeling.
One of these advantages is that multi-shot modeling can be performed more efficiently in the
frequency domain than in the time domain, and another is the ability to work on a
frequency-by-frequencbya sis,w hich makesi t possiblet o distributef requenciesa crossp rocessors.
Consideringt hat frequency-domainm odeling is popular in waveform inversionb ecauseo f source
wavelet estimationa nd multi-shot modeling, 3D frequency-domainfi nite-elementm odelingc an be
effectively used in 3D waveform inversion. We derive a numerical dispersion relationship for the
3D frequency-domainfi nite-elementm ethoda nd then analyzen umericald ispersiono n the basiso f
dispersion curves. From the dispersion analysis, we determine th€ minimum number of grid points
per wavelength. The validity of the 3D finite-element modelirtg algorithm is examined for a
three-layeredm odel and the SEG/EACE salt model.
KEY WORDS:3D modeling, acousticw ave equation,f requency-domainf,i nite-elemem,
numerical dispersion
- 초록
Ha, T., Choi, Y., Shin, C.S. and Min, D.-J., 2009. Numerical modeling for 3D acoustic wave
equation in the frequency domain. Joumal of Seisnic Exploration, l8t 57-19.
We investigatea frequency-domainfi nite-elementm ethod for three-dimensionaml odeling
of the acousticw ave equation.F requency-domaimn odelingh ass everala dvantageos ver time-domain
modeling,e ven though it requiresh ugec omputationaml emoryc omparedt o time-domainm odeling.
One of these advantages is that multi-shot modeling can be performed more efficiently in the
frequency domain than in the time domain, and another is the ability to work on a
frequency-by-frequencbya sis,w hich makesi t possiblet o distributef requenciesa crossp rocessors.
Consideringt hat frequency-domainm odeling is popular in waveform inversionb ecauseo f source
wavelet estimationa nd multi-shot modeling, 3D frequency-domainfi nite-elementm odelingc an be
effectively used in 3D waveform inversion. We derive a numerical dispersion relationship for the
3D frequency-domainfi nite-elementm ethoda nd then analyzen umericald ispersiono n the basiso f
dispersion curves. From the dispersion analysis, we determine th€ minimum number of grid points
per wavelength. The validity of the 3D finite-element modelirtg algorithm is examined for a
three-layeredm odel and the SEG/EACE salt model.
KEY WORDS:3D modeling, acousticw ave equation,f requency-domainf,i nite-elemem,
numerical dispersion
More