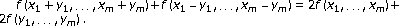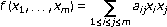Papers
p-adic interpolating function associated with Euler numbers
Taekyun Kim , Daeyeoul Kim & Ja Kyung Koo | Journal of Nonlinear Mathematical Physics 14/2 (2007)
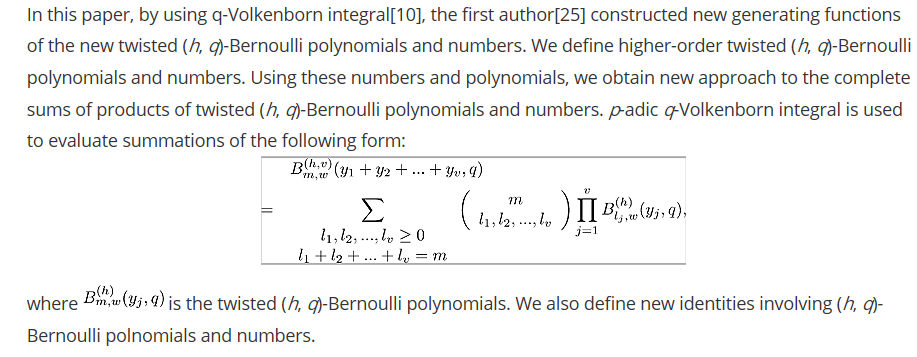
New approach to the complete sum of products of the twisted (h,q)-Bernoulli numbers and polynomials
Kurt,V.,김대열,Simsek,Y. | J. Nonlinear Math. Phys. 14/1 (2007)
On a cubic equation and a Jensen-quadratic equation
Jae-Hyeong Bae and Won-Gil Park | Abstract and Applied Analysis 2007 (2007)
A multi-dimensional functional equation having quadratic forms as solutions
Won-Gil Park and Jae-Hyeong Bae | Journal of Inequalities and Applications 2007 (2007)
On exact convergence rate of strong numerical schemes for stochastic differential equations
Dougu Nam | Bulletin of the Korean Mathematical Society 44/1 (2007)
On the infinite products derived from theta series I
김대열,Ja Kyung Koo | J. Korean Math. Soc. 44/1 (2007)
A functional equation originating from quadratic forms
Jae-Hyeong Bae and Won-Gil Park | Journal of Mathematical Analysis and Applications 326/2 (2007)
Inverse shadowing in Geometric Lorenz flows
Taeyoung Choi,Manseob Lee | Journal of the chungcheong mathematical society 20/4 (2007)
Kwan-Sun Yoon,Chi-Ok Hwang and Taeyoung Won | Journal of the Korean Physical Society 50/6 (2007)
Compact adjoint operators and approximation properties
Ju Myung Kim | Journal of Mathematical Analysis and Applications 327 (2007)