Papers
The path topology and the causal completion
Do-Hyung Kim | Journal of Mathematical Physics 47/7 (2006)
Understanding Representational Sensitivity in the Iterated Prisoners Dilemma with Fingerprints
E.Y. Kim,,N. Leahy,D. Ashlock | IEEE Transactions on Systems, Man, and Cybernetics, Part C (Applications and Reviews) 36/4 (2006)
Geodesic connectedness of the causal completion in the Lorentzian geometry
Jin-Whan Yim,Do-Hyung Kim | General Relativity and Gravitation 38/3 (2006)
Some nonlinear wave equations with memroy source term and acoustic boundary conditions
Jung Ae Kim,Jong Yeoul Park | Numerical Functional Analysis and Optimization 27/7-8 (2006)
Jung Ae Kim,Jong Yeoul Park | Mathematical Methods in the Applied Sciences 29/9 (2006)
Generalization of characterizations of the trigonometric functions
Y.-S. CHUNG and S.-Y. CHUNG,J.-H. KIM | Math. Proc. Cambridge Philos. Soc. 141/3 (2006)
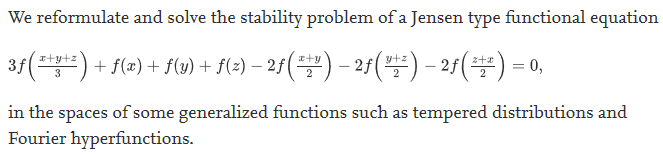
Stability of a Jensen type equation in the space of generalized functions
Y.-S. CHUNG and S.-Y. CHUNG,J.-H. Kim | J. Math. Anal. Appl. 321/6 (2006)
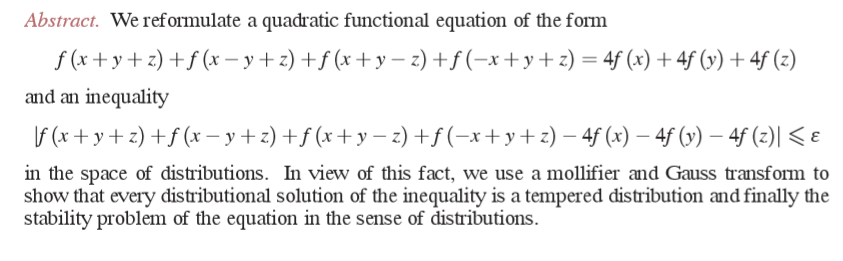
Stability of quadratic functional equation in the space of distributions
Y.-S. CHUNG and S.-Y. CHUNG,J.-H. KIM | Math. Inequal. Appl. 9/2 (2006)
Taeyoung Ha,Sukjoon Pyun and Changsoo Shin | Journal of Computational Physics 214/1 (2006)
Taeyoung Ha,Sangwon Seo and Dongwoo Sheen | Computer Modeling in Engineering & Sciences 14/1 (2006)